23 June 2004
The language of music
A recent article in Nature talks about a paper written by an Argentinian physicist named Damian Zanette in which he relates musical compositions to written (or spoken) texts. His theory is that note frequency (the number of occurrences of each note) in music matches the word frequencies found in linguistic expression. Zanette found a relationship between the number of different notes and each note's number of occurrences. He based his research on a linguistic property called Zipf's law.
Zipf's law states the frequency of use of the nth-most-frequently-used word in any natural language is approximately inversely proportional to n.
That basically means that if the word "the" appears 20 times (occurrence) and is the 10th most frequent word (rank), then the 100th most frequent word will appear only two times. In his paper, Zanette plots the words in David Copperfield by occurrence and rank and gets a graph with a straight line.
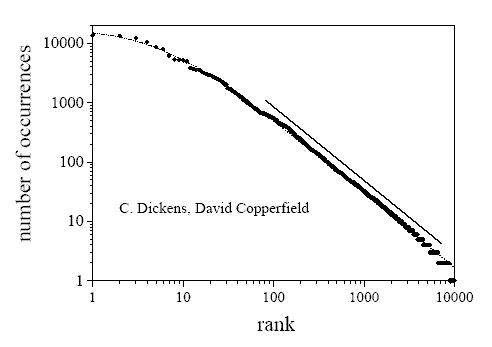
I've also heard Zipf's law called the power law, but the premise is the same. Zanette then plots graphs for four piano compositions from different time periods:
- Prelude No. 6, Das Wohltemperierte Klavier, J. S. Bach,
- First movement, Allegro, from the Sonata in C (K. 545), W. A. Mozart,
- Second movement, Menuet, from the Suite Bergamasque, Claude Debussy,
- The first of Three Piano Pieces (Op. 11, No. 1), Arnold Schoenberg
He plots each note of the compositions by occurrence and rank and gets results similar to those of textual analysis.

- The vinyl and the concert posted by sstrader on 21 May 2016 at 1:59:26 PM
- Rod McKuen posted by sstrader on 22 March 2016 at 8:58:35 PM
- More political transcriptions posted by sstrader on 20 March 2016 at 10:19:06 AM
- Notes on We Don't Care About Music Anyway posted by sstrader on 7 June 2015 at 1:06:34 PM
- Ives Concord Sonata posted by sstrader on 13 February 2015 at 5:13:17 PM